GRADE
11
Physics SPH3U
This course develops students' understanding of the basic concepts of physics. Students will study the laws of dynamics and explore different kinds of forces, the quantification and forms of energy (mechanical, sound, light, thermal, and electrical), and the way energy is transformed and transmitted. They will develop scientific-inquiry skills as they verify accepted laws and solve both assigned problems and those emerging from their investigations. Students will also analyze the interrelationships between physics and technology, and consider the impact of technological applications of physics on society and the environment. Prerequisite: Grade 10 Science SNC2D (Academic)
TABLE OF CONTENTS
Kinematics
Scalar and Vector
What is the main difference between scalars and vectors ?
Solution
Scalar measurements have only have a magnitude (no direction). Vectors have both magnitude and direction.
For example 45 meters West is a vector quantity.
For example 45 meters West is a vector quantity.
The following list consists of scalar and vector quantities. Determine which are the four scalar quantities.
Solution
Scalars: Distance, Time, Mass, Speed...
Scalar quantities do not have direction, and consist only of a magnitude.
Scalar quantities do not have direction, and consist only of a magnitude.
The following list consists of scalar and vector quantities. Determine which are the four vector quantities.
Solution
Vector quantities have both direction and magnitude.
The three vectors are velocity, displacement, position, and acceleration. (Force is another vector, not shown here).
The three vectors are velocity, displacement, position, and acceleration. (Force is another vector, not shown here).
Calculating Average Speed
An Ironman® triathlon is a race consisting of three events: swim, bike, and run. If Sarah McSpeedy completed each event with the distances and times in the following table, calculate her average speed in the race, in m/s. Solution
| Event | Distance (km) | Time (min) |
| Swimming | 3.86 | 76 |
| Cycling | 180 | 385 |
| Running | 42.2 | 294 |
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Convert kilometers to meters, and minutes to seconds:
Total distance divided by total time:
Calculating Average Speed
A car travels around a circular track with an average speed of 216 km/hour. If the time to travel around the track 10 times is 837.76 s, determine the radius of the track. (Circumference = 2r)
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Convert speed units into m/s: 216 km/hr ÷ 3.6 = 60 m/s
The circumference is the distance: multiply by 10 to get the total distance.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
The circumference is the distance: multiply by 10 to get the total distance.
Average and Instantaneous Speed
It is possible for the instantaneous speed to be much higher or lower than the average speed.
Solution
Instantaneous speed is the speed at a precise moment in time. Since the average speed is an average that can result from many different instantaneous speeds, then the instantaneous speed can be a vary wide range of values lower or higher than the average speed calculated.
For example if you were to walk most of the distance to some place and sprint the last 10 meters, then the average speed would likely be much lower than the instant you were sprinting the fastest speed.

For example if you were to walk most of the distance to some place and sprint the last 10 meters, then the average speed would likely be much lower than the instant you were sprinting the fastest speed.
 |
Distance Vs. Displacement
A marathon runner is training and runs from one end of the Golden Gate Bridge, and back. If the bridge is 7,180 m long, what is the 16 year-old's total displacement? Solution
 |

Distance is the total length (7,180m + 7,180m), while displacement is the change in position (+7,180m - 7,180m). Since the runner returns to the starting position, the change in position is zero.
Calculating Average Velocity
A cat chases its tail in a circle with a radius of 0.25 m for a period of 10.0 s. If the cat ends up at the exact place as it started, the cat's average velocity equals zero.
Solution

Average Velocity is a change in position divided by time, and depends on the total displacement, rather than the distance. If the change in position equals zero, then the average velocity also equals zero.
Types of Motion
Throwing a ball straight up into the air, and then catching it on the way back down is best described as what type of motion?
Solution
This is non-uniform motion because there is acceleration.
Which of the following is non-uniform motion?
Solution
Remember that acceleration is non-uniform motion, so the soccer ball has non-uniform motion.
If the acceleration of an object is zero, then the object must be at rest.
Solution
Acceleration can be zero at rest or in constant motion. For example if a jogger runs at a constant 9 km/hr, then acceleration = 0.
Which of the following scenarios displays an object that is speeding up in the positive direction.
Solution
Since the motion is defined as being in the positive direction, then the velocity is positive.
"Speeding up" in the positive direction indicates that there is positive acceleration.
(If the object was "slowing down" in the positive direction, then the acceleration would be negative).
"Speeding up" in the positive direction indicates that there is positive acceleration.
(If the object was "slowing down" in the positive direction, then the acceleration would be negative).
Non-Uniform Motion: Horizontal
A car traveling at 100 m/s decelerates at a rate of 4.0 m/s2.
How long (in seconds) will it take the car to stop?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
The initial velocity is +100 m/s, the acceleration is -4.0 m/s2, and the final velocity is 0 m/s. Find time:
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Find the distance (in meters) that the car travels during deceleration?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
The initial velocity is +100 m/s, the acceleration is -4.0 m/s2, final velocity is 0 m/s, and time is 25 s. Find distance:
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Kinematics and Mass
A bowling ball with a mass of ten times the mass of a golf ball, is dropped from rest at the same time as the golf ball, from the same height. Neglecting air resistance, the bowling ball will hit the ground first because it is heavier.
Solution
Mass does not matter, the both the bowling and golf balls will hit the ground at the same time. None of the kinematics equations have mass in them. They will fall with the same time have the same final velocity on impact at the ground.
Gravity is the acceleration causing the motion of the two balls, and assuming this is on Earth, then it is the same for both balls.
Gravity is the acceleration causing the motion of the two balls, and assuming this is on Earth, then it is the same for both balls.
Non-Uniform Motion: Vertical
An object is thrown vertically upward. It has a speed of 12.2 m/s when it has reached half of its maximum height.
What is the maximum height reached?
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Use 12.2 m/s as your initial velocity and the final velocity = 0 m/s
This distance calculated will be one-half of the maximum height.
Givens:
v1 = 12.2 m/s
v2 = 0 m/s
a = -9.8 m/s2
calculate d:
Since this is half the maximum height, then the maximum height is 3.1m
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
This distance calculated will be one-half of the maximum height.
Givens:
v1 = 12.2 m/s
v2 = 0 m/s
a = -9.8 m/s2
calculate d:
Since this is half the maximum height, then the maximum height is 3.1m
How long will it take to reach its maximum height?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Use the maximum height of 3.1 m
Givens:
v2 = 0 m/s
a = -9.8 m/s2
d = 3.1m
Find time (t)
The total time is 0.8 seconds.
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
v2 = 0 m/s
a = -9.8 m/s2
d = 3.1m
Find time (t)
The total time is 0.8 seconds.
What was the object's initial velocity?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
v2 = 0 m/s
a = -9.8 m/s2
t = 0.8 s
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
v2 = 0 m/s
a = -9.8 m/s2
t = 0.8 s
Calculating Uniform Acceleration (Non-Uniform Motion)
A car traveling at 10 m/s South accelerates 5 m/s2 South over a 5.0 s time interval. Determine the final velocity of the car.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [S]
Hint Unavailable
Givens:
v1 = 10 m/s [S]
a = 5 m/s2 [S]
t = 5.0 s
Find v2
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [S]
Hint Unavailable
v1 = 10 m/s [S]
a = 5 m/s2 [S]
t = 5.0 s
Find v2
A car travelling at 10 m/s [W] comes to a complete stop with an acceleration of -10 m/s2 [W]. Determine how long this takes.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
v1 = 10 m/s [W]
v2 = 0 [W]
a = -10 m/s2 [W]
Find t
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
v1 = 10 m/s [W]
v2 = 0 [W]
a = -10 m/s2 [W]
Find t
Motion Graphs
The slope on a position-time graph represents:
Solution
Video
Position is a vector quantity.
The area (underneath the plotted line or curve) on a velocity-time graph represents:
Solution
Video
Displacement is a vector quantity.
The slope on a velocity-time graph represents:
Solution
Video
The area (underneath the plot) on an acceleration-time graph represents:
Solution
Video
Motion Graph Calculations
Determine the average velocity of the motion in the following position-time graph from 0s - 15s.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Velocity is a vector quantity that involves displacement.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Velocity is a vector quantity that involves displacement.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Describing Motion Graphs
At what time(s) is velocity equal to zero?
Solution

Velocity is zero where the y-axis indicates 0 m/s, which is located at 16 seconds.
Describing Motion Graphs
In which portion(s) of the graph is the magnitude of acceleration, uniform and non-zero?
Solution

• The slope on a velocity-time graph represents the acceleration.
• The flat line in phase I indicates a slope of zero, so the acceleration is zero.
• Phase II has a negative slope, which is negative acceleration.
• Phase III has a positive slope, which is positive acceleration.
• Phase IV is a curve, which is a non-uniform acceleration as it constantly changes and would have different instantaneous accelerations along the curve.
• The flat line in phase I indicates a slope of zero, so the acceleration is zero.
• Phase II has a negative slope, which is negative acceleration.
• Phase III has a positive slope, which is positive acceleration.
• Phase IV is a curve, which is a non-uniform acceleration as it constantly changes and would have different instantaneous accelerations along the curve.
Motion Graph Calculations
Determine the average acceleration of the motion in the following velocity-time graph from 0s - 12s.
Solution
Video
 Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Average acceleration is just from the first point at 0s to the last point at 12s.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Average acceleration is just from the first point at 0s to the last point at 12s.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Motion Graph Calculations
Determine the displacement of the motion in the following velocity-time graph from 0s - 16s.
Solution
Video
 Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
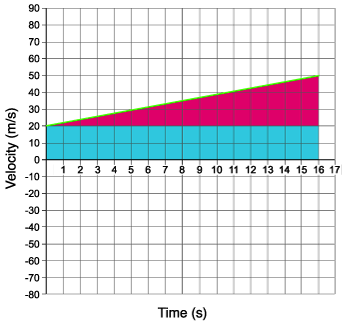
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable

Motion Graph Calculations
Determine the change in velocity of the motion in the following acceleration-time graph from 1s - 9s.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Change in velocity = area under acceleration time function.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Change in velocity = area under acceleration time function.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Getting Used to North, East, South, West
People walking with a compass heading of [E 20˚ S], would be equivalent to:
Solution

Drawing a sketch of the compass heading on a N-E-S-W system shows that the two headings overlap and are the same: [S 70˚ E] and [E 20˚ S].
Determine the resultant velocity of the velocities: 25 km/hr [N 50˚ E], 30 km/hr [S 40˚ E], and 60 km/hr [W].
Break into components...
Average Velocity
In one hour, a boat travels 6 km North, then 4 km East, and then 3 km South. The average velocity is:
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
km/hr [N 53˚ E]
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
km/hr [N 53˚ E]
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
km/hr [N 53˚ E]
Hint Unavailable
Relative Velocity
A person is walking 1 m/s East, on a subway car that is travelling 20 m/s West. Determine the relative velocity of the person with respect to the ground.
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [West]
Hint Unavailable
Here I choose to define West as positive, and East as negative.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [West]
Hint Unavailable
Relative Velocity
A river flows at 2.3 m/s [N 43˚ W]. A kayak heads [N 23˚ W] at 3.6 m/s relative to the water. What is the velocity of the kayak with respect to the ground?
Solution
v =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [N 31.2˚ E]
Hint Unavailable
Do a sketch and determine that the obtuse angle = 23˚ + 90˚ + (90˚ - 43˚) = 160˚
Use the cosine law to solve for the resultant, which is the longest side length in the triangle in your sketch.
Givens:
b = 3.6 m/s
c = 2.3 m/s
A = 160˚
Solve for the direction using sin law (given side-side-angle)...
43˚ - 11.8˚ = 31.2˚
Therefore the velocity = 6 m/s [N 31.2˚ E]
v =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [N 31.2˚ E]
Hint Unavailable
Use the cosine law to solve for the resultant, which is the longest side length in the triangle in your sketch.
Givens:
b = 3.6 m/s
c = 2.3 m/s
A = 160˚
Solve for the direction using sin law (given side-side-angle)... 43˚ - 11.8˚ = 31.2˚
Therefore the velocity = 6 m/s [N 31.2˚ E]
Kinematics Equations (Non-Uniform Motion, Uniform Acceleration)
Solve the following questions without using acceleration.
If an object accelerates from 2 m/s to 22 m/s over 5.0 s, determine the distance travelled.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Givens:
v1 = 2 m/s
v2 = 22 m/s
t = 5.0 s
Find d
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
v1 = 2 m/s
v2 = 22 m/s
t = 5.0 s
Find d
A bike accelerates over 0.5 km for 1 minute 40 seconds. Determine the initial speed if the final speed is 3.6 km/hr.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
d = 0.5 km = 500m
t = 1:40 min = 100s
v2 = 3.6 km/hr ÷ 3.6 = 1.0 m/s
Find v1
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
d = 0.5 km = 500m
t = 1:40 min = 100s
v2 = 3.6 km/hr ÷ 3.6 = 1.0 m/s
Find v1
Kinematics Equations (Non-Uniform Motion, Uniform Acceleration)
Solve the following questions without using distance.
Determine the acceleration if something speeds up from 12 m/s to 32 m/s over 10 s.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Givens:
v1 = 12 m/s
v2 = 32 m/s
t = 10 s
Find
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
v1 = 12 m/s
v2 = 32 m/s
t = 10 s
Find
A racecar driver accelerates uniformly at 5 m/s2 over 15s to a final speed of 100 m/s. Determine the initial speed.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
a = 5 m/s2
t = 15 s
v2 = 100 m/s
Find v1
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
a = 5 m/s2
t = 15 s
v2 = 100 m/s
Find v1
Kinematics Equations (Non-Uniform Motion, Uniform Acceleration)
Solve the following questions without using initial velocity.
An object accelerates over a distance of 839.5 m at 3 m/s2 [E] in 23 s. Find the final velocity.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [E]
Hint Unavailable
Givens:
= 3 m/s2 [E]
t = 23s
d = 839.5m
Find
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s [E]
Hint Unavailable
= 3 m/s2 [E]
t = 23s
d = 839.5m
Find
An object travelling at an unknown initial velocity comes to rest over a displacement of 73.5 m [W]. If the acceleration is 3 m/s2, determine the time this takes.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
d = 73.5m [W]
a = 3 m/s2
= 0
Find t
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
d = 73.5m [W]
a = 3 m/s2
= 0
Find t
An object accelerates at 6 m/s2 over 85.0 m to a final velocity of 2 m/s. Determine the time this takes.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
d = 85.0m
a = 6 m/s2
= 2 m/s
Find t
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
d = 85.0m
a = 6 m/s2
= 2 m/s
Find t
Kinematics Equations (Non-Uniform Motion, Uniform Acceleration)
Solve the following questions without using final velocity.
Find the initial velocity of an object that accelerates 5 m/s2 over 52m in 4s.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
= 5 m/s2
d = 52m
t = 4s
Find
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
= 5 m/s2
d = 52m
t = 4s
Find
Determine the time for an object to accelerate 3 m/s2 from rest over 37.5 m.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
= 3 m/s2
d = 37.5m
= 0 m/s
Find t
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
= 3 m/s2
d = 37.5m
= 0 m/s
Find t
Kinematics Equations (Non-Uniform Motion, Uniform Acceleration)
Solve the following questions without using time.
Determine the displacement of an object that accelerates at 4 m/s2 [S] from an initial velocity of 15 m/s [S] to a final velocity of 23 m/s [S].
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m [S]
Hint Unavailable
Givens:
= 4 m/s2 [S]
= 15 m/s [S]
= 23 m/s [S]
Find
Hint
Clear
Info
Incorrect Attempts:
CHECK
m [S]
Hint Unavailable
= 4 m/s2 [S]
= 15 m/s [S]
= 23 m/s [S]
Find
An object accelerates at 3.2 m/s2 over 85m. Determine the initial velocity if the final velocity is 34.2 m/s.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
= 3.2\ m/s2
d = 85m
= 34.2\ m/s
Find
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
= 3.2\ m/s2
d = 85m
= 34.2\ m/s
Find
Theory: Gravity and Galileo
Two objects of same size but different mass are dropped from a tower at the same initial speed. Ignore air resistance.
Solution
Ignoring air resistance, the acceleration of falling objects (any masses) is the same because the acceleration (gravity) is the same, 9.81 m/s2 [Down] and the initial velocities are the same.
As you can see in the equation below, the final velocities of the different masses would be the same (because initial velocity, acceleration, and displacement are the same) so the masses would hit the ground at the same time.
As you can see in the equation below, the final velocities of the different masses would be the same (because initial velocity, acceleration, and displacement are the same) so the masses would hit the ground at the same time.
 |
Gravity and g's
An acceleration of [↓] equals
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 [↓]
Hint Unavailable
In this case, g does not represent grams, rather it represents the unit of gravity, -9.81 m/s2.
So would be
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 [↓]
Hint Unavailable
So would be
Projectile Motion: Combining Uniform and Non-Uniform Motion due to Gravity
A baseball is thrown horizontally off a cliff and takes 4.0 s to reach the ground. It travels a distance of 25 m from the base of the cliff.
 |
How high is the cliff where the ball was thrown?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
The vertical component of the motion of the baseball has the same time as the whole projectile motion. So we will solve for height based on the purely vertical component of the motion.
Givens:
v1 y = 0 m/s
t = 4 s
a = -9.81 m/s2
Find height, h
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Givens:
v1 y = 0 m/s
t = 4 s
a = -9.81 m/s2
Find height, h
Determine final velocity of the baseball in the horizontal x-direction.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
In the horizontal (x) component direction--motion is uniform, or constant velocity:
Givens:
dx = 25 m
t = 4 s
Find vx
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
dx = 25 m
t = 4 s
Find vx
Determine final velocity of the baseball in the vertical y-direction.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
In the vertical (y) component direction:
(Note that the time is the same in the x and y components)
Givens:
v1 y = 0 m/s
t = 4 s
a = -9.81 m/s2
Find v2 y
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
(Note that the time is the same in the x and y components) Givens:
v1 y = 0 m/s
t = 4 s
a = -9.81 m/s2
Find v2 y
Determine the total velocity of the baseball at the instant before it hits the ground.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
This question uses values calculated previously (final velocity components in the 'x' and 'y' direction). You must calculate these (not shown here) if you haven't already, hence the significance of this question. Use vector addition of the x and y velocities found previously. Use pythagorean theorem:
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Non-Uniform Motion: Inclined Planes
A box slides down an inclined plane from rest, with uniform acceleration and attains a speed of 20 m/s in 6.0 s. Assume no friction.
Determine the acceleration of the box.
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Givens:
v1 = 0 m/s
v2 = 20 m/s
t = 6.0 s
Find a
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
v1 = 0 m/s
v2 = 20 m/s
t = 6.0 s
Find a
What will the speed of the box be 4s after it began its descent?
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Givens:
v1 = 0 m/s
a = 3.3 m/s2
t = 4.0 s
v2 = ?
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
v1 = 0 m/s
a = 3.3 m/s2
t = 4.0 s
v2 = ?
How far down the plane will the box be at the end of 4s?
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Givens:
v1 = 0 m/s
a = 3.3 m/s2
t = 4.0 s
d = ?
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
v1 = 0 m/s
a = 3.3 m/s2
t = 4.0 s
d = ?
Forces
Mass and Weight
The S.I. unit of mass to use in physics calculations is the:
Solution
Kilogram
In physics, the quantities mass and weight
Solution
Weight = Mass × Gravity
Newtons's Laws of Motion
Newton's first law states
Solution
Commonly known as the law of inertia. Newton's first law in his own words, translated from Latin to English: "Law I: Every body persists in its state of being at rest or of moving uniformly straight forward, except insofar as it is compelled to change its state by force impressed."
A space shuttle launch depends on the propulsion of massive amounts of rocket fuel propellant to produce upward thrust on the shuttle as the hot gases are expelled. Which of Newton's laws explains this best?
Solution
Newton's third law. For every action, there is an equal and opposite reaction
Which of Newton's laws best explains why car drivers should always wear a seatbelt to protect them from harm?
Solution
A body will continue in motion unless acted upon by a net force, the seatbelt providing the force holding the occupant back at the same motion as the car. In other words, the person's body would continue moving forward into harm without the force of the seatbelt holding it back.
A 10,000 kg comet collides head-on with a 100kg satellite.
Solution
Newton's third law. The collision involves two different masses colliding in opposite directions. Even though the masses are different, the objects will experience equal and opposite forces.
Newtons's Laws of Motion: Net Force
Determine which of the following scenarios has a net force equal to zero.
Solution
Constant motion (or zero motion) involves a balance of forces. For the skiier their normal force would balance with the force due to gravity (if we neglect friction).
Which of Newton's laws best explains why skydivers reach a constant (terminal) velocity during freefall?
Solution
Newton's first law: an object stays at rest or in constant motion unless acted upon by a net force. Terminal velocity occurs when there is no net force because the force due to gravity is equal and opposite to the force of friction of the air (Newton's third law).
Newtons's Laws of Motion: Simple Net Force Applications
Two teams are competing in a tug-of-war. If one team pulls with a combined force of 5000N East, and the other team pulls with combined force of -5001N West, what is the net force in the rope?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
N [West]
Hint Unavailable
The forces are opposite in direction, but are not equal magnitudes. Therefore there is a net force of -1 Newton West.
Hint
Clear
Info
Incorrect Attempts:
CHECK
N [West]
Hint Unavailable
Unfortunately a 500 year old Oak tree has rotted and died in the community and is being taken down over public safety concerns. A truck attaches a cable to the trunk to topple the tree and pulls with a force of 3200N South. The tree doesn't budge. A second, bigger truck comes to the rescue and attaches another cable pulling 5800N West. What is the net force of the two cables on the tree truck?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
N [S 61.1˚ W]
Hint Unavailable
Vector addition of the two forces, using pythagorean theorem since the two forces are perpendicular... = 6624 N
Hint
Clear
Info
Incorrect Attempts:
CHECK
N [S 61.1˚ W]
Hint Unavailable
Newtons's Laws of Motion: Force, Mass, and Acceleration
Choose the correct proportionality between force, mass, and acceleration.
Solution
F = m • a
Increasing acceleration (with constant mass) increases the force.
Increasing mass (with constant force) decreases the acceleration.
Increasing acceleration (with constant mass) increases the force.
Increasing mass (with constant force) decreases the acceleration.
An object with mass 8m is accelerated with a net force 4F. If the same force is applied to a smaller mass, 2m, then the new acceleration would be:
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
times higher
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
times higher
Hint Unavailable
Free Body Diagrams
Draw the following free body diagrams.
a) An apple hanging from a tree branch.
Solution
Show the force due to gravity (Fg) downwards and the force of tension (FT) in the apple stem upwards.
A sled being pulled at constant velocity, with a rope angle of 30˚ to the horizontal.
Solution
The sled has a normal force (FN) downwards, the rope has a tension force (FT) in the rope due equal to the applied force (not shown). There is also the force due to gravity (Fg) downwards on the sled.
A block of bricks being accelerated upwards by the cable of a crane.
Solution
Force due to gravity (Fg) downwards on the bricks and tension force (FT) up in the crane cable.
A box of cookies sliding down an incline at a constant speed.
The last car of a train being accelerated forwards by the car in front, with friction.
A skier skidding to a stop in the middle of an inclined hill.
The Geometry of Net Force
Determine the net force in each of the horizontal and vertical directions in following examples.
Solution
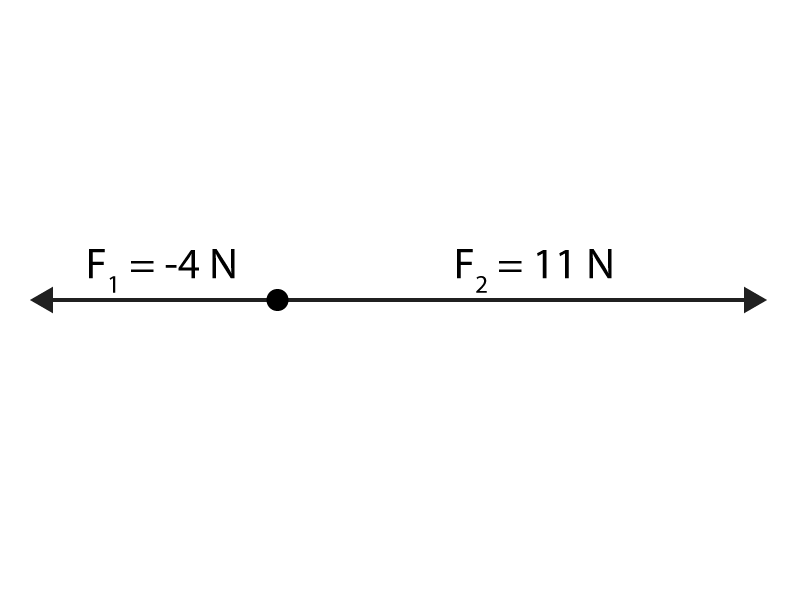 Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
(FNET y = 0 N)
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
(FNET y = 0 N)
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Solution
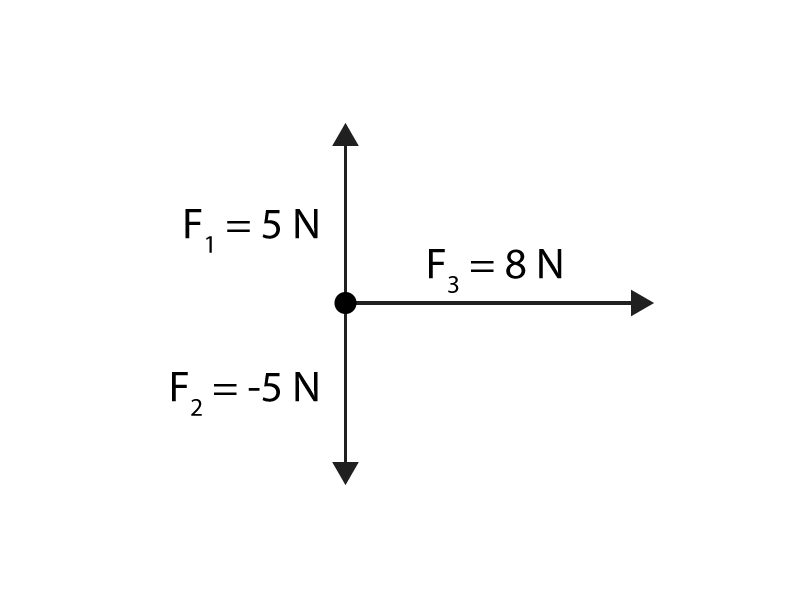 Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Solution
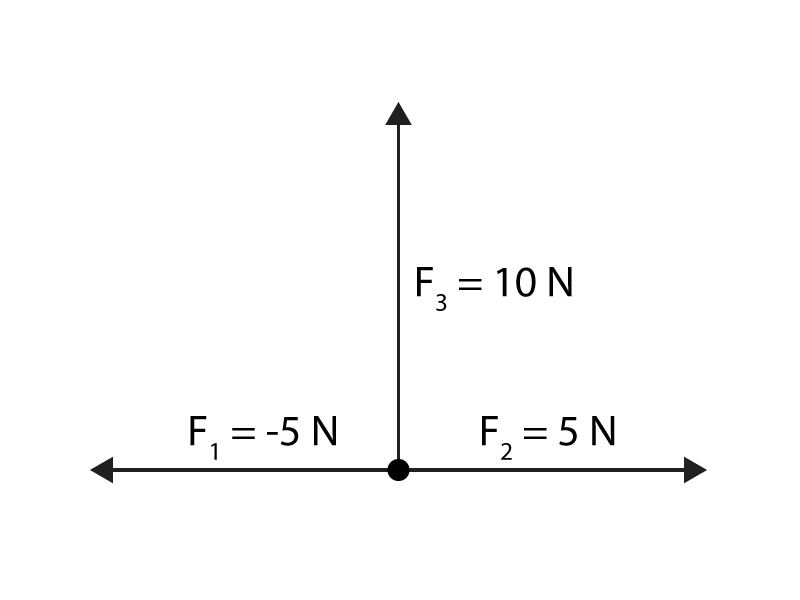 Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Solution
 Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Solution
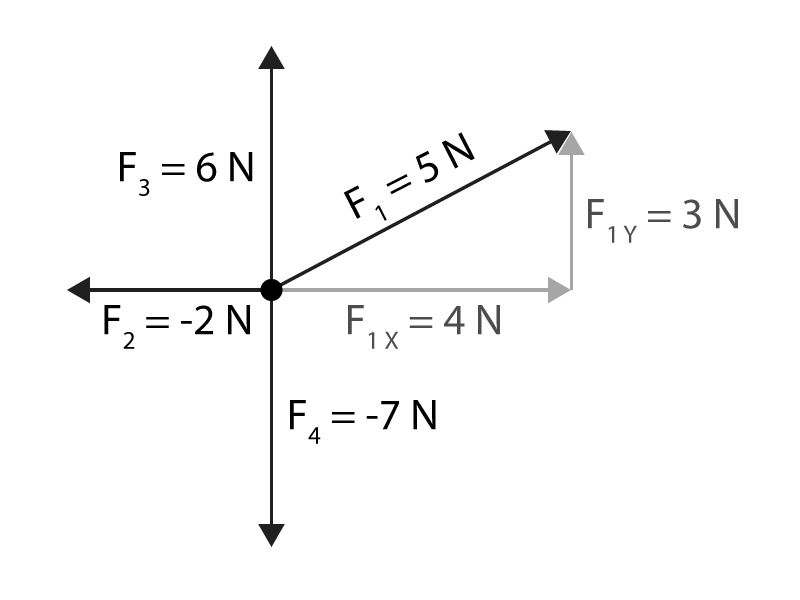 Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
FNET x = N FNET y = N
Incorrect Attempts:
CHECK
Hint Unavailable
Simple Force Intro
The force required to accelerate a frictionless, 1000 kg rollercoaster from rest to 80 m/s in 10 seconds is:
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
F = ma = ... = 8000 N
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Tension and Angles
Someone pulling a sled at an angle of 60˚ along a flat, frictionless surface pulls so hard that the 10.0 kg sled momentarily loses contact with the ground. Determine the minimum pulling force necessary to do this.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
To lift upward, off the ground, the 'y' component of the force tension opposes the force due to gravity
Think about the two opposite and parallel forces that are opposing,
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
To lift upward, off the ground, the 'y' component of the force tension opposes the force due to gravity
A special hauling rig designed to haul away the last NASA space shuttle is designed to pull a 20,000kg shuttle on a frictionless track. The hauling rig has a rope attached to the shuttle that makes an angle of 15˚ to the horizontal. If the net acceleration of the shuttle is 0.60 m/s2 horizontally, determine the tension force exerted by the rope on the shuttle.
Solution
F =
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Givens:
m = 20,000 kg
anet (x) = 0.60 m/s2
Find Ftension
Since the net acceleration is in the x-direction, first calculate the net force in the x-direction with:
Then calculate the net force in the length of the rope...
F =
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
m = 20,000 kg
anet (x) = 0.60 m/s2
Find Ftension Since the net acceleration is in the x-direction, first calculate the net force in the x-direction with: Then calculate the net force in the length of the rope...
Coefficients of Friction µk
The coefficients of kinetic (µk) and static (µs) friction do not have units.
Solution
The equation Ff = Fµ × FN shows that the units of force cancel (Newton), leaving behind the unit-less coefficient of friction.
Forces
A 400 kg motorcycle traveling at 10 m/s accelerates forward with an applied force of 2000 N.
How long will it take the motorcycle to reach 40 m/s if the force of friction is 90 N?
Solution
Video
t =
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
m = 400 kg
v1 = 10 m/s
FA = 2000 N
V2 = 40 m/s
Ff = 90 N
Write an equation for net force in the horizontal direction.
Given the net force, determine the acceleration (using Newton's 2nd law).
Now using kinematics, calculate the time.
t =
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
m = 400 kg
v1 = 10 m/s
FA = 2000 N
V2 = 40 m/s
Ff = 90 N Write an equation for net force in the horizontal direction. Given the net force, determine the acceleration (using Newton's 2nd law). Now using kinematics, calculate the time.
A car with the same mass applying the same force accelerates at 0.5 m/s2. Determine the force of friction on the car.
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Givens:
m = 400 kg
a = 0.5 m/s2
FA = 2000 N
First determine the net force with friction...
Then using Newton's 2nd law, with FNET find the Force of friction...
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
m = 400 kg
a = 0.5 m/s2
FA = 2000 N First determine the net force with friction... Then using Newton's 2nd law, with FNET find the Force of friction...
Forces
Determine the force required to accelerate a 0.10 kg hockey puck from 1.0 m/s to 60 m/s over 0.25 s.
Solution
F =
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Givens:
m = 0.10 kg
v1 = 1.0 m/s
v2 = 60 m/s
t = 0.25 s
Find F
Calculate acceleration first:
Then calculate net force:
F =
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
m = 0.10 kg
v1 = 1.0 m/s
v2 = 60 m/s
t = 0.25 s
Find F Calculate acceleration first: Then calculate net force:
Forces
Your physics teacher stands on a scale in an elevator to demonstrate the effect of acceleration on weight. At rest, the scale reads 80 kg, going downwards the scale reads 60kg. Determine the acceleration of the elevator.
Solution
a =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 down
Hint Unavailable
Givens:
m(actual) = 80 kg
m(observed) = 60 kg
Find a
The observed mass on the scale is an indication of the reactive Normal Force (FN). At rest the normal force equals the force due to gravity. When the elevator accelerates downward the normal force equals:
(Side note: an elevator accelerating upward has a normal force = Fg + Fa)
a =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 down
Hint Unavailable
m(actual) = 80 kg
m(observed) = 60 kg
Find a The observed mass on the scale is an indication of the reactive Normal Force (FN). At rest the normal force equals the force due to gravity. When the elevator accelerates downward the normal force equals: (Side note: an elevator accelerating upward has a normal force = Fg + Fa)
Forces
A rescue helicopter airlifts a 200 kg moose that was trapped in the middle of a thin sheet of ice on a lake. The only cable available in the helicopter can support a maximum force of 3000 N. Determine the maximum acceleration the helicopter can rise upwards.
Solution
a =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 up
Hint Unavailable
Givens:
m = 200 kg
Fmax = 3000 N
Find amax
The tension in the cable is based on the force due to gravity of the moose and the applied force of the acceleration of the helicopter.
a =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2 up
Hint Unavailable
m = 200 kg
Fmax = 3000 N
Find amax The tension in the cable is based on the force due to gravity of the moose and the applied force of the acceleration of the helicopter.
Forces With Friction
A 1.0 kg block of sandpaper is pushed at a constant speed over wood with a force of 11.0 N. Determine the coefficient of kinetic friction between the wood and the sandpaper in this situation.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Forces With Friction
A 100 kg cube pallet of bricks in the back of a 2000 kg pickup truck. Accelerating from rest, the driver notices that the load of bricks starts to slide dangerously when the truck reaches an acceleration of ±0.37 m/s2.
Determine the coefficient of static friction between the cube pallet and the bed of the pickup truck.
Solution
µs =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Givens:
mbricks = 100 kg
mtruck = 2000 kg
v1 = 0 m/s
a = ±0.37 m/s2
Find Ff
At the maximum point of acceleration, the force of static friction of the cube pallet of bricks is equal to the net force of the pickup truck:
µs =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
mbricks = 100 kg
mtruck = 2000 kg
v1 = 0 m/s
a = ±0.37 m/s2
Find Ff At the maximum point of acceleration, the force of static friction of the cube pallet of bricks is equal to the net force of the pickup truck:
Describe what the person could do to the brick load to be able to accelerate faster in the pickup truck.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
See that increasing the mass of the bricks would increase the acceleration...
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Forces With Friction
You accelerate your Porsche at 14.72 m/s2 on a perfectly level surface without spinning out the wheels. Assuming drag and kinetic friction is negligible, what is the coefficient of static friction between the tire treads and the road surface?
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Static Friction of Rolling Car Tire
The force of static friction of a car tire is in the same direction as the movement of the car.
Solution
True, this is what allows the car to drive forward... force of static friction and direction of car acceleration are in the same direction.
Static Friction
A conveyor belt is moving a box along a flat surface. The box slips as it gets moving and then becomes stationary on the moving conveyor belt. During this time the forces of static and kinetic friction are always in the same direction as the movement of the conveyor belt.
Solution
The forces of static and kinetic friction carry the box forward... same direction.
Forces
An Amazon™ fulfillment center sorts packages by routing them onto different conveyor belt tracks. The conveyor belts move at a constant 2.0 m/s and the coefficient of kinetic friction of 0.66 exists between the conveyor belt surface and a package. When packages are lowered onto the conveyor belt from rest, how long does it take for a package to stop sliding?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
Givens:
v1 = 0 m/s
v2 = 2 m/s
µk = 0.66
Find time
Force due to friction is in the same direction as the net force on the package - they are equal. First get acceleration with net force equation:
Now that you have acceleration, calculate the time with kinematics:
Hint
Clear
Info
Incorrect Attempts:
CHECK
s
Hint Unavailable
v1 = 0 m/s
v2 = 2 m/s
µk = 0.66
Find time Force due to friction is in the same direction as the net force on the package - they are equal. First get acceleration with net force equation: Now that you have acceleration, calculate the time with kinematics:
Inclined Planes
Increasing the angle of inclination on an inclined plane will decrease the weight.
Solution
Weight (mg) is constant.
For a stationary object, increasing the angle of inclination on an inclined plane will:
Solution
Weight and the coefficient of static friction are constant. The force of friction increases.
Inclined Planes with Friction
A block rests on the side of a hill that is angled at 10˚ to the horizontal. Calculate the coefficient of static friction, µs.
Solution
Video
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Inclined Planes Without Friction
A world-class skier can ski down a 60˚ hill with negligible friction. Determine the skier's acceleration down the hill.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s2
Hint Unavailable
A 20 kg sled is being pulled up a hill inclined at 10˚ to the horizontal. The sled is pulled by a string with a tension force of 10.0 N that makes an angle of 20˚ with the surface of the inclined plane. Determine the normal force of the sled.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
N
Hint Unavailable
Force of Gravity
The force of gravity (Fg = mg) is an approximation for the force of gravity on an object near the surface of the Earth.
Solution
True
Gravitational acceleration, g is also known as gravitational field strength.
Solution
True, at the Earth's surface, it is approximated as roughly -9.81 m/s2.
Newton's Law of Universal Gravitation
Newton's law of universal gravitation states that, anywhere in the universe, the force of gravity between two objects is directly proportional to the product of the masses of the two objects, and inversely proportional to the square of the distance between their centers of mass.
Solution
True
What equation is used for the force of gravity, Fg at altitudes high above the Earth's surface?
Solution
Make sure to use Newton's Law of Universal Gravitation at altitudes high above the Earth's surface, and not the simplification (Fg = mg)
Gravitation Equations
Which of the following is the formula used for determining gravitational field strength, g?
Solution
The units of gravitational field strength (g) are ms-1 or Nkg-1.
Gravitation
Using the constants below, determine the force of gravity on a 20,000kg space station orbiting 200 km above the surface of the Earth.
Solution
massEarth = 5.98 × 1024 kg
radiusEarth = 6.4 × 106 m
Gravitational constant, G = 6.67 × 10-11 Nm2/kg2
Fg =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
N
Hint Unavailable
Fg =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
N
Hint Unavailable
Force of Gravity Theory
An interplanetary space shuttle is launched from Earth to a planet with three times the mass of the Earth, and half the radius. Compared to the force of gravity on Earth, the force of gravity on the other planet is:
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
times higher
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
times higher
Hint Unavailable
Energy, Work, and Power
Forms of Energy
Which of the following is not a form of energy?
Solution
Temperature is not a form of energy, but heat is.
A ray of light travels from the Sun to the Earth through the vacuum of space with what form of energy?
Solution
Radiation is the only form of energy that can travel through a vacuum (space without matter).
 |
Work Units
True or false?
Work = ∆ Energy
What is the unit typically used for work?
Work is a scalar quantity (rather than a vector quantity).
Solution
A scalar is a magnitude without a direction.
Work SI Base Units
Use the equation for work due to gravity to derive the equivalent in base units (kg, m, s).
Solution
W = Fg · d
Work
Determine the mass of an object that can be lifted a height of 350 cm, with an energy of 5000 J.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
kg
Hint Unavailable
350cm = 3.5m
Hint
Clear
Info
Incorrect Attempts:
CHECK
kg
Hint Unavailable
Work on an Incline
A cart is being pushed with a net 10 N force up a frictionless incline (parallel to the face of the incline) that has a vertical distance of 40 m, and horizontal distance of 30 m, and a ramp length of 50 m. Determine the work necessary to push the cart to the top of the incline.
Solution
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Use the displacement in the same direction as the force. Work is conservative, meaning the magnitude does not depend on the path taken.
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Work
Calculate the height a 10 kg object can be lifted on the moon (moon's gravity = 1.6 m/s2) if the object can be lifted 1,000 cm on earth with the same energy.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
1,000cm = 10m
moon's gravity = 1.6 m/s2
earth's gravity = 9.8 m/s2
Work = (Force) × Distance = (Mass × Gravity) × Distance.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
moon's gravity = 1.6 m/s2
earth's gravity = 9.8 m/s2
Work = (Force) × Distance = (Mass × Gravity) × Distance.
Work
A strong person can lift and hold a 50 kg stone at waist-height, 1m above the ground. Determine the work done by the strong person to hold the stone there for 10 minutes.
Solution
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
While there is a vertical force (Fg = mg), there is no displacement parallel to this force.
The stone does not move, displacement = 0
Work is the energy applied by an applied force over a change in position. Work is zero when the displacement parallel to the force is zero.
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
The stone does not move, displacement = 0 Work is the energy applied by an applied force over a change in position. Work is zero when the displacement parallel to the force is zero.
Work Theory
Work equals zero in which of the following situations?
Solution
- Work due to gravity on a hockey puck sliding with constant velocity across a frictionless surface.
- Work done on an object that experiences no forces.
- Work due to the normal force of a computer resting on the flat surface of a table.
W = F · d
- Work equals zero when the distance direction is not parallel to the force direction. Force of gravity is vertical while the distance travelled is horizontal, or the distance in the same direction is zero, so work is zero.
- Force equals zero, so work equals zero.
- Distance equals zero, so work equals zero.
- Work due to gravity on a hockey puck sliding with constant velocity across a frictionless surface.
- Work done on an object that experiences no forces.
- Work due to the normal force of a computer resting on the flat surface of a table.
- Work equals zero when the distance direction is not parallel to the force direction. Force of gravity is vertical while the distance travelled is horizontal, or the distance in the same direction is zero, so work is zero.
- Force equals zero, so work equals zero.
- Distance equals zero, so work equals zero.
Mechanical Energy
 |
An apple is dropped from a height of 10 m. The maximum speed of the apple occurs when the height equals zero.
Which of the following is correct?
A ball is thrown into the air. As the ball rises the magnitude of mechanical energy does not change.
Solution
Mechanical energy is constant the whole way because it is always a sum of gravitational potential and kinetic energies.
The apple is held 10.0 m above the ground with a total mechanical energy of 10.0 J. Determine the gravitational potential energy and kinetic energy at the points in the following table.
Solution
Hint
Clear
Info
Height Gravitational Potential Energy Kinetic Energy Total Mechanical Energy
10.0 m J J 10 J
5.0 m J J 10 J
0 m J J 10 J
Incorrect Attempts:
CHECK
Hint Unavailable
total mechanical energy = gravitational potential energy + kinetic energy
Height Gravitational Potential Energy Kinetic Energy Total Mechanical Energy
10.0 m 10 J 0 J 10 J
5.0 m 5 J 5 J 10 J
0 m 0 J 10 J 10 J
Hint
Clear
Info
| Height | Gravitational Potential Energy | Kinetic Energy | Total Mechanical Energy |
| 10.0 m | J | J | 10 J |
| 5.0 m | J | J | 10 J |
| 0 m | J | J | 10 J |
Incorrect Attempts:
CHECK
Hint Unavailable
| Height | Gravitational Potential Energy | Kinetic Energy | Total Mechanical Energy |
| 10.0 m | 10 J | 0 J | 10 J |
| 5.0 m | 5 J | 5 J | 10 J |
| 0 m | 0 J | 10 J | 10 J |
Mechanical Energy
A 200 g ball rolls 2.0 m/s off a table, which is 1.2 m above the ground.
Determine the kinetic energy at the moment the ball leaves the tabletop.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
200 g = 0.2 kg
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Determine the gravitational potential energy at the moment the ball leaves the tabletop. [Use g = 9.8 m/s2]
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
200 g = 0.2 kg
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Total mechanical energy = 2.75 J
Solution
total mechanical energy = gravitational potential energy + kinetic energy
= 2.35 J + 0.4 J
= 2.75 J
= 2.35 J + 0.4 J
= 2.75 J
The maximum speed of the ball is:
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
The maximum speed occurs at the ground, when total mechanical energy is 0% gravitational potential energy and 100% kinetic energy.
The maximum speed occurs at the ground, when total mechanical energy is 0% gravitational potential energy and 100% kinetic energy. Set total mechanical energy equal to kinetic energy: solve for v:
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
The maximum speed occurs at the ground, when total mechanical energy is 0% gravitational potential energy and 100% kinetic energy.
Work Due to Friction on an Incline Plane
A 10 kg box slides down an incline at a constant velocity from a height of 12 m. The coefficient of kinetic friction of the surface is 0.2 and the plane is inclined at 50˚ to the horizontal.
The work due to friction on an incline plane is calculated with the following equation, where h is the vertical height in meters.
Solution
Workfriction = Forcefriction × height

Works is nonconservative, but it must use the distance, d of the face of the plane that the box slides on.
Workfriction = Forcefriction × distance
Workfriction = Forcefriction × distance
Calculate the work due to friction.
Solution
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Energy Conservation
A 500 kg roller-coaster car is perched 20.0 m above ground, at the start of a steep drop.
The following three quantities are equal in magnitude.
| Potential Energy at the Top |
| Kinetic Energy at the Bottom |
| Total Mechanical Energy |
Assuming the track is frictionless, find the speed at the bottom of the drop.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Total mechanical energy equals 9.8 × 104 J.
Solution
Speed at the bottom (v) calculated previously using conservation of energy.
Can be calculated with PEtop
Can be calculated with PEtop
Calculate how high the roller-coaster car would roll up a flat incline on the other end of the track if the track is angled at 60˚ to the horizontal and the coefficient of kinetic friction on the incline is 0.20
Solution
 h =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Work due to friction uses the incline distance = height/(sin60˚)
h =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Work due to friction uses the incline distance = height/(sin60˚)
h =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Work due to friction uses the incline distance = height/(sin60˚)
Energy Conservation
A roller-coaster goes around a loop in the track with a maximum height 50m above the ground, at a speed of 20 m/s. It then goes around a second loop at 30 m/s.
Is the maximum height of the second loop greater, or less than the maximum height of the first loop?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
The maximum height of the second loop is less than the first because the roller-coaster is moving faster due to the conservation of total mechanical energy.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Calculate the maximum height of the second loop.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
The total mechanical energy (TME) of loop 2 equals the total mechanical energy (TME) of loop 1.
TME = KE + PE
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
TME = KE + PE
Conservation of Energy and Efficiency
Determine the law of conservation of energy.
A student pedals a bicycle on the ground with a total of 400 J of energy. If the student and the bicycle combined weigh 80 kg, and the speed is 3 m/s, determine the percent efficiency of this situation.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
%
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
%
Hint Unavailable
 |
Power
A television uses 100 W of power over 60 minutes. Calculate the energy consumed.
Solution
E =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
E = (Power)(time) = (100 W)(3600 s) = 360,000 J
E =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Power
An elevator weighs 8000 kg when fully loaded and must travel a height of 80 m from the ground to the top floor of a building. The company needs the elevator to make it to the top in 20 seconds.
 |
Determine the minimum power of the motor that must be used, assuming 100% efficiency.
Solution
P =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
W
Hint Unavailable
P =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
W
Hint Unavailable
If the efficiency of the motor was 75%, then the power of the motor used should be 25% higher than the theoretical power calculated in the previous section.
Determine the minimum power of the motor that must be used, assuming 75% efficiency.
Solution
P =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
W
Hint Unavailable
(In reality the chosen motor should be considerably more powerful than this).
P =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
W
Hint Unavailable
Energy & Power
Determine the longest run time of the electric-assisted bicycle setups below.
Solution
Energy = Wh, (Watt·hours)
Power = W, (Watt)
Power = W, (Watt)
Two AA batteries in series, provides 3.0 Volts. If each battery cell is rated at 2.8 Ah (and the total is 2.8 Ah), determine the total energy in kJ. [1 Wh = 3.6 kJ]
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
kJ
Hint Unavailable
First determine the watt-hours 'Wh'
Enegy,
Hint
Clear
Info
Incorrect Attempts:
CHECK
kJ
Hint Unavailable
Power and Velocity
The following derivation is correct.
A frictionless hockey puck travels at 10 m/s when a force of 50 N is applied to it. Determine the power applied.
Solution
P =
Hint
Clear
Info
Incorrect Attempts:
CHECK
W
Hint Unavailable
P =
Hint
Clear
Info
Incorrect Attempts:
CHECK
W
Hint Unavailable
Heat Energy
Heat is the measure of energy in something.
Solution
Heat is the transfer of energy from a higher to lower temperature.
Temperature is the measure of the average kinetic energy of molecules in a substance.
Temperature is the measure of the average kinetic energy of molecules in a substance.
Heat Transfer
State the four methods of heat transfer from a flame on a stovetop to the inside of an egg boiling in a pot of water.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
1) Convection and radiation from the flame through the air
2) Conduction through the metal
3) Convection through the water
4) Convection through the egg if it's liquid, convection if it's solid.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
2) Conduction through the metal
3) Convection through the water
4) Convection through the egg if it's liquid, convection if it's solid.
Specific Heat Capacity
Specific heat capacity is the same for all substances.
Solution
Specific heat capacity is different for all different substances.
Substances with higher specific heat capacities can hold more heat for a given amount of mass.
The units of specific heat capacity (c) can be J/g˚C or J/kgK.
Solution
Heat Exchange
An increase in heat is indicated as:
Solution
+ q, in relation to the energy input into the system.
Zeroth (0th) Law of Thermodynamics
The zeroth law of thermodynamics states that if two objects are in thermal equilibrium with a third object, then the two objects are also in thermal equilibrium with eachother.
Solution
Thermal equilibrium occurs when the temperatures are the same (average molecular kinetic energies are the same) and there is no net transfer of heat energy.
Thermal Energy
Substances with the same temperature have the same thermal energy.
Solution
Thermal energy depends on the size of the substance. For example, it is easy for a "cold" swimming pool to contain more thermal energy than a hot cup of tea. Besides size, the other factor that matters is the type of substance.
Kelvin and Celsius
A 100 K increase in temperature is equal to
Solution
While the change is the same,
∆K = ∆˚C
The magnitudes are not the same,
K ≠ ˚C
Specific Heat Capacity
Specific heat capacity (c) of silver is 0.24 J/g˚C
q = mc∆T100 J of heat is lost from a 2.5 kg sample of silver with an initial temperature of 10˚C. Find the final temperature.
Solution
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
Heat lost is negative, therefore q = -100J
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
If the same 10˚C block of silver was placed into a 1000 kg pool of water at 10˚C, determine the heat (q) of the silver.
Solution
Heat is the movement, or transfer of energy between things with different temperatures. If the temperature is the same (10˚C), then there is no energy transfer. Therefore there is no heat exchange.
Specific Heat Theory
If the specific heat of aluminum is 2.01 J/(g·˚C), how much heat is required to raise the temperature of a 2 g block by 1˚C?
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
4.02 J
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
Specific Heat: q = m c ∆T
100 J of heat is added to a 25 g unknown block at 5.45˚C causing the block to heat to 10.00˚C. Determine the identity of the unknown sample.
Specific Heat: q = m c ∆T
You want to make ice tea in the summertime by brewing a pot of 1.0L (1.0kg) of tea at 372K, and cooling it to the drinking temperature of 275K. You have an unlimited supply of 50g ice cubes at 263K. Determine the number of ice cubes to bring the ice tea to the perfect temperature. (cwater = 4.18 J·g-1·˚C-1, cice = 2.1 J·g-1·˚C-1, Hf (water) = 373 J/g)
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
cubes
Hint Unavailable
Since the tea and ice cubes reach the same temperature, the heat lost in the tea equals the heat gained in the ice cube.
Let the total mass of ice cubes be 50n (where 'n' is the number of ice cubes, and each cube is 50g)
Therefore (rounding up), a minimum of 21 ice cubes are needed.
Hint
Clear
Info
Incorrect Attempts:
CHECK
cubes
Hint Unavailable
Let the total mass of ice cubes be 50n (where 'n' is the number of ice cubes, and each cube is 50g) Therefore (rounding up), a minimum of 21 ice cubes are needed.
Specific Heat, 2 Samples, Find mass: q = m c ∆T
The laboratory scale is broken and a chemist needs to figure out the mass of a known sample of pure silver. The chemist has a heat source, calorimeter, and thermometer to use so they decide to find the mass using calorimetry. Starting at room temperature, 25˚C, the sample place in the calorimeter, immersed in 0.45kg of water (4.18 J·g-1·˚C-1). It takes 142,875 J of heat to bring the sample to a boil at 100˚C. Given the specific heat capacity of silver is 0.240 J·g-1·˚C-1, determine the mass of the silver.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
g
Hint Unavailable
Make sure to plug in 450 g for water, and not 0.45kg
Hint
Clear
Info
Incorrect Attempts:
CHECK
g
Hint Unavailable
Specific Heat, 2 Samples, Find Tf: q = m c ∆T
Equal masses of ethanol (2.46 J·g-1·˚C-1) and water (4.18 J·g-1·˚C-1) are mixed in a 700g solution at room temperature, 298K. Find the final temperature of the mixture when 124,031.88 J of heat is applied.
Solution
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
K
Hint Unavailable
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
K
Hint Unavailable
Specific Heat, 2 Samples, Find Tf Mass Ratio: q = m c ∆T
A 1,050 ˚C glowing-hot block of gold (0.129 J·g-1·˚C-1) is placed in a calorimeter filled with nothing but air (1.02 J·g-1·˚C-1) at room temperature, 298K. If the mass of gold is 5 times the mass of air, find the final temperature in the calorimeter assuming no heat loss.
Solution
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
IMPORTANT: gain in heat = +q, loss of heat = -q
Tf =
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
Waves and Sound
Types of Waves
You need to know the main types of oscillations in waves.
Which is not a type of oscillation (periodic motion)?
Solution
The 3 types of oscillation are:
- Longitudinal
- Transverse
- Torsional
- Longitudinal
- Transverse
- Torsional
A guitar string behaves as what type of wave?
Solution
Transverse waves have amplitude perpendicular to the direction of travel...
A sound wave behaves as what type of wave?
Solution
Longitudinal waves oscillate with amplitude parallel to the direction of travel.
Types of Waves
Particles in longitudinal waves oscillate parallel to the direction of travel, while particles in transverse waves oscillate perpendicular to the direction of travel of the wave.
Solution
For a longitudinal wave, imagine a sound wave traveling horizontally. The air particles also oscillate horizontally.
For a transverse wave, imagine a boat ('particle') stalled in the ocean with very large waves. The waves travel horizontally across the surface of the water, while the boat travels vertically.
For a transverse wave, imagine a boat ('particle') stalled in the ocean with very large waves. The waves travel horizontally across the surface of the water, while the boat travels vertically.
Wave Terminology
Given the diagram below,

Which of the following is not one wavelength.
Solution
All the answer choices measure out one wavelength.
Name the points in the table.
Solution
Hint
Clear
Info
Point Name
B or F
D or H
vertical height of A to B, or C to D
A
Incorrect Attempts:
CHECK
Hint Unavailable
Point Name
B or F Crest
D or H Trough
vertical height of A to B, or C to D Amplitude
A Node
Hint
Clear
Info
| Point | Name |
| B or F | |
| D or H | |
| vertical height of A to B, or C to D | |
| A |
Incorrect Attempts:
CHECK
Hint Unavailable
| Point | Name |
| B or F | Crest |
| D or H | Trough |
| vertical height of A to B, or C to D | Amplitude |
| A | Node |
SI Units of Waves
What is the SI unit for frequency?
Solution
Frequency is inversely proportional to period (s), while the SI unit hertz (Hz) is used in place of s-1.
Frequency and Period
An object that oscillates many times per second with a short wavelength has a high frequency.
Frequency and wavelength are inversely proportional, or reciprocals.
An object oscillating in simple harmonic motion completes 30 cycles in 2.75s. Calculate the frequency Solution
Pendulum Oscillation
A pendulum begins oscillating at point A.

Where is the equilibrium position?
Solution
The equilibrium is always located in the center of the oscillation.
What is the point of maximum displacement?
Solution
Maximum displacement occurs at the furthest points from the equilibrium position. The maximum displacement is also known as the 'amplitude'
What is the point of maximum velocity?
Solution
Maximum velocity always occurs at the equilibrium position.
What is the point of maximum acceleration?
Solution
Maximum acceleration occurs at the two points of maximum displacement (or amplitude), where the pendulum is changing the direction vector.
Wave Speed Theory
For a wave traveling in air...
What affects the speed of a longitudinal (e.g. sound) or transverse (e.g. light) wave?
Solution
Many people think that frequency affects speed, but only wavelength affects the speed of a wave that is in existence.
This is for a wave traveling in a medium that has a certain frequency (for sound, think pitch). The speed of this wave can change depending on the medium, but the frequency (or sound, pitch) stays the same, while the wavelength changes.
v = ƒ λ
This is for a wave traveling in a medium that has a certain frequency (for sound, think pitch). The speed of this wave can change depending on the medium, but the frequency (or sound, pitch) stays the same, while the wavelength changes. v = ƒ λ
As a light wave enters a less dense (slower for light, faster for sound) medium, what happens?
Solution
For a fixed frequency, a slower speed (v), would result in a decrease in wavelength (λ): v = ƒ λ
As a light wave enters a more dense (faster for light, slower for sound) medium, what happens?
Solution
For a fixed frequency, a faster speed (v), would result in an increase in wavelength (λ): v = ƒ λ
An upright wave travels from a fast medium to a slow medium, what happens the reflected wave at the medium boundary?
Solution
When waves travel from a slow to fast medium, part of the wave is reflected backwards from the medium boundary and is upright, or positive.
When waves travel from a fast to slow medium, part of the wave is reflected backwards from the medium boundary and is inverted, or negative.
(Actually, the positive and negative aspect depends on the incident orientation of the wave).
When waves travel from a fast to slow medium, part of the wave is reflected backwards from the medium boundary and is inverted, or negative.
(Actually, the positive and negative aspect depends on the incident orientation of the wave).
When a wave enters a medium with a different density, some energy of the wave is directed backwards in the opposite direction of travel.
Sound Wave Travel
Sound can travel in a vacuum (space).
Solution
Sound is a longitudinal wave. Longitudinal waves require matter (particles) to oscillate and propagate energy.
The speed of sound is fastest in which of the following materials?
Solution
The speed of sound is fastest in dense materials like iron. (Aside: the speed of sound in iron is approximately 17 times faster than in air).
air at 20˚C is 343m/s, while iron is 5950m/s, sea water is 1530 m/s
Note that the speed of light behaves opposite to sound; light slower in denser mediums (think air versus diamond).
air at 20˚C is 343m/s, while iron is 5950m/s, sea water is 1530 m/s
Note that the speed of light behaves opposite to sound; light slower in denser mediums (think air versus diamond).
String/Spring Wave Travel
For a string or a spring, the speed (v) equals:
- v = speed, (m/s)
- = linear density, (kg/m)
- FT = Tension, (N)
And linear density equals:
- m = mass, (kg)
- L = length, (m)
For a string with length 1.0 m, mass 50 g, determine the wavelength of a wave with frequency 90 s-1, if the force of tension in the string is 200 N.
Solution
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Substitute the linear density equation into the wave speed equation,
And set that equal to the wave equation,
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
For a wave in a string, the linear density (µ) is doubled, and the force of tension is tripled. Determine the factor of change in the speed, rounded to the nearest tenth decimal.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
times
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
times
Hint Unavailable
Period of a Pendulum
The equation for period of a pendulum (T), where l is length in meters, and g is gravity, in m/s2.
The period of an oscillating pendulum depends on the mass attached to the end and the height the pendulum is pulled-back and released from.
Solution
Notice that mass and height are both not included in the equation for period of a pendulum, therefore mass and height do not have an effect the period of a pendulum.
The only factors that affect the period of a pendulum are length and gravity.
The only factors that affect the period of a pendulum are length and gravity.
If a simple pendulum has a period of 2.0 s, determine the length of the pendulum.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
g = 9.81 m/s2
Rearrange, isolate, and solve for length, l.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Rearrange, isolate, and solve for length, l.
Wave Behavior
An ocean wave encounters a small opening. As it passes through the small opening the wave undergoes:
Solution
Diffraction is the bending of a wave as it passes through a narrow opening or passes by an obstacle.
An ocean wave travels from deep water onto a shallow reef. As it passes the deep-shallow boundary, the wave undergoes:
Solution
Refraction is the bending of a wave as it enters a medium with a different density.
Principle of Superposition
Which of the following would result in destructive interference between two waves?
For total destructive interference to occur between two waves, what must be the same?
- Amplitude
- Frequency
- Wavelength
- ½λ Path Difference
- Amplitude
- Frequency
- Wavelength
- ½λ Path Difference
Resonance
For resonance to occur in an object, a periodic driving force must be in contact with that object.
Solution
Resonance can occur at a separation distance, when objects are not in contact. (Note that: mechanical resonance must have the resonating object in contact with the driving force).
Describe how resonance occurs
Solution
For an object to resonate, a periodic driving force that has the same frequency as the natural frequency of an object must exist.
A 450 Hz tuning fork is made to oscillate near the following objects. Which of the following will start to experience vibrations?
Standing Waves
A standing wave in a string oscillates with a speed of 1 m/s.
Standing waves are the result of an interference pattern.
Determine the distance between two adjacent nodes in a standing wave, in terms of wavelength, λ (lambda).
If two nodes are spaced 0.4 m apart, determine the frequency of the wave.
Solution
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
v = 1 m/s
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
Beat Frequency
A 1,000 Hz tuning fork harmonizes with a second tuning fork, creating a 300 Hz beat frequency. Calculate the pitch (frequency) of the second tuning fork.
Solution
Solve the absolute value equation like this...
See that there are two possible answers...
See that there are two possible answers...
Frequency and Length
The frequency in a standing wave on a string is 800 Hz.
Determine the new frequency if the length of the string is doubled.
Solution
f' =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
frequency, f is inversely proportional to length, L.
Doubling the length will decrease the frequency by a factor of ½... = 400 Hz
f' =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
Doubling the length will decrease the frequency by a factor of ½... = 400 Hz
If this new frequency is the 4th harmonic, determine the fundamental frequency, f1.
Solution
f1 =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
The nth harmonic has frequency fn = v/λn = (n)v/2L = (n)f1
1st, Fundamental frequency f1 = v/λ1 = (1)v/2L = f1
2nd harmonic has frequency f2 = v/λ2 = (2)v/2L = 2f1
3rd harmonic has frequency f3 = v/λ3 = (3)v/2L = 3f1
4th harmonic has frequency f4 = v/λ4 = (4)v/2L = 4f1
f1 =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
1st, Fundamental frequency f1 = v/λ1 = (1)v/2L = f1
2nd harmonic has frequency f2 = v/λ2 = (2)v/2L = 2f1
3rd harmonic has frequency f3 = v/λ3 = (3)v/2L = 3f1
4th harmonic has frequency f4 = v/λ4 = (4)v/2L = 4f1
Frequency
Standing waves in strings, and waves in open-end pipes share the same formula for frequency, where n is an integer {1, 2, 3, ...}
Solution
Closed-end pipes use a different formula, where n is an odd integer: {1, 3, 5, ...}
Open and Closed Pipes
Closed pipes (closed on one end, open at the other end) have antinodes at the close end.
Solution
Closed pipes have nodes at the closed end and antinodes at the open end.
An open pipe (open at both ends) has antinodes at both ends.
Solution
Antinodes are located at open ends.
Open and Closed Pipes
Open pipes have the following resonant lengths (where lambda, λ is one wavelength):
Solution
¼λ, ¾λ, 1¼λ
Closed pipes have these resonant lengths.
Open pipes have:
½λ, λ, 1½λ
Open pipes have: ½λ, λ, 1½λ
Closed-End Air Columns (Closed Pipes)
The table below shows the relationship between harmonic number and wavelength for closed pipes/tubes/columns.
| Harmonic | Amount of Wavelength in Column | Length (m) | Wavelength (m) |
| 1 | |||
| 3 | |||
| 5 | |||
| 7 |
There is no such thing as a second harmonic in closed-end columns. The second resonant length in a closed-end column is ¾λ.
Solution
Second harmonics are found in open-end columns.
A wave in a closed-end pipe has a 4.0 m wavelength at the 2nd resonant length. Determine the length of the pipe.
Solution
L =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
wavelength = 4.0 m
2nd resonant length = 3rd harmonic
L =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
2nd resonant length = 3rd harmonic
Speed, Wavelength, and Frequency
A tweeter speaker plays a 2,000 Hz note with a 0.1725 m wavelength at the same time that the connected subwoofer plays a 90 Hz note. Calculate the wavelength of the subwoofer sound wave.
Solution
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
You know that the speed of a sound wave depends on the temperature of the air. If the temperature is the same then the speed is the same.
First calculate the speed of sound
Since the speaker is in the same room with the same air temperature, then the speed is the same for the subwoofer wave...
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
First calculate the speed of sound Since the speaker is in the same room with the same air temperature, then the speed is the same for the subwoofer wave...
Speed, Wavelength, and Frequency
The wavelength of a certain frequency of light is 5.0 × 10-7 m in air, what is its frequency? (vlight = 3 x 108m/s).
Solution
f =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
f =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
Calculations with Waves
A pendulum travels left and right for a total of 20 m, and completes 4 cycles, determine the amplitude.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Convert the distance travelled for 4 cycles, into the distance for 1 cycle:
Realize that the amplitude is ¼ of the total distance of a cycle.
Therefore the amplitude is 1.25 m
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Calculations with Waves
A wave in the ocean travels 15 m in 3.0 s and the distance between adjacent minimum points is 10 m.
Determine the frequency of the wave.
Solution
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
wavelength = 10 m.
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
It takes 20 s for the same ocean wave to pass by a surfer, reflect off a barricade, bouncing back to her. Calculate the distance from the surfer to the barricade.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
v = 5 m/s
t = 20 s
20 s represents twice the distance; there-and-back.
The barricade is 50 m from her.
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
t = 20 s
20 s represents twice the distance; there-and-back. The barricade is 50 m from her.
Determine the number of wave cycles that pass a fixed surfing point in the water over the course of 15 seconds.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
cycles
Hint Unavailable
ƒ = 0.5 Hz
t = 15 s
(This is actually a bit high for real life but w/e)
Hint
Clear
Info
Incorrect Attempts:
CHECK
cycles
Hint Unavailable
t = 15 s (This is actually a bit high for real life but w/e)
Calculations with Waves
The 1st and 5th antinodes in a wave are 18 m apart. A total of 12 peaks pass through a point over the course of 4 seconds.
Determine the wavelength of the wave.
Solution
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Each adjacent antinode is ½λ.
λ =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m
Hint Unavailable
Determine the speed of the wave.
Solution
v =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
λ = 9 m
v =
Hint
Clear
Info
Incorrect Attempts:
CHECK
m/s
Hint Unavailable
Speed of Sound in Air
Determine what will happen to the speed of sound as a wave travels from warm air to denser, cool air, given the equation below, where T is the temperature, in ˚C.
Solution
The frequency of pitch will go down and it will sound lower as the speed decreases.
Calculate the air temperature in ˚C if the speed of sound in air is 359 m/s
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
˚C
Hint Unavailable
Frequency and String Length
A note on a 80 cm guitar string is 250 Hz.
The following ratios for frequency 'f' and length 'l' are correct.
Solution
Yes, you can reciprocate both sides of the equation.
Determine the frequency if the same string oscillates at 70 cm.
Solution
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
Givens:
l1 = 80cm
f1 = 250Hz
l2 = 70cm
Find f2
f =
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hz
Hint Unavailable
l1 = 80cm
f1 = 250Hz
l2 = 70cm
Find f2
Intensity and Surface Area
You are located 50m from the speakers at a concert where the intensity is 0.0008 W/m2. You decide to move closer to the stage and you are 5.0 m away from the speakers. Calculate the new intensity. Solution
 |
Hint
Clear
Info
Incorrect Attempts:
CHECK
w/m2
Hint Unavailable
You know that the sound dissipates in a spherical shape, with the distance, being radius, r...
Givens:
I1 = 0.0008 W/m2
I2 = ?
r1 = 50 m
r2 = 5.0 m
You also know that the power of the speaker system is constant.
Givens:
I1 = 0.0008 W/m2
I2 = ?
r1 = 50 m
r2 = 5.0 m
You also know that the power of the speaker system is constant.
Sound and Intensity
The intensity (W/m2) of the background noise in a restaurant, 60dB, is increased by a factor of 1,000. Determine the magnitude of the new intensity level in dB, by inspection. Solution
 |
i =
Hint
Clear
Info
Incorrect Attempts:
CHECK
dB
Hint Unavailable
The intensity changes by a factor of ×10 when the decibels change by +10.
1000 = 103 = 10 × 10 × 10... The change is + 30 dB.
Therefore the new intensity is 60 dB + 30 dB = 90 dB
(90 dB is like the noise level of a food blender)
1000 = 103 = 10 × 10 × 10... The change is + 30 dB.
Therefore the new intensity is 60 dB + 30 dB = 90 dB
| Intensity (W/m2) | decibels (dB) |
| 10-12 | 0 |
| 10-11 | 10 |
| 10-10 | 20 |
| 10-9 | 30 |
| 10-8 | 40 |
| 10-7 | 50 |
| 10-6 | 60 |
| 10-5 | 70 |
| 10-4 | 80 |
| 10-3 | 90 |
| 10-2 | 100 |
| 10-1 | 110 |
| 100 = 1 | 120 |
| 101 | 130 |
| ... | ... |
Calculate how many times louder a 100 dB sound is than a 50 dB sound.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
times louder
Hint Unavailable
Hint
Clear
Info
Incorrect Attempts:
CHECK
times louder
Hint Unavailable
Calculate the dB intensity of a sound 100 times louder than 70 dB.
Solution
Hint
Clear
Info
Incorrect Attempts:
CHECK
dB
Hint Unavailable
Solve for the higher intensity, dBH
When solving for a variable in the exponent, take the log of both sides of the equation. This will drip the exponent, multiplied down in-front...
Hint
Clear
Info
Incorrect Attempts:
CHECK
dB
Hint Unavailable
Electricity and Magnetism
Chemistry in Physics
Which statement is not correct?
Solution
Charged (positive or negative) atoms are called ions.
Charging an Object
An object is always positively charged when there are a high number of positively charged particles within the object.
Solution
Not necessarily. An object with a neutral charge might have a high number of positively charged particles within it, but with an equal number of negatively charged particles.
An object is positively charged when:
(number of positive charges) > (number of negative charges)
An object is positively charged when: (number of positive charges) > (number of negative charges)
The Electrostatic Series
When copper is rubbed against fur, what will happen? Solution
| Attraction of Electrons | Material |
| High attraction | Copper |
| ↕ | Ebonite |
| ↕ | Plastic |
| ↕ | Fur |
| ↕ | Glass |
| Low attraction | Acetate |
Flow of electrons only.
Charging
Determine the charge remaining on the object, if any.
A positively charged rod is brought close to a neutral, grounded object. While the rod and object are in close proximity, the grounding is removed from the object, then the rod is removed.
Solution
The positively charged rod will attract the negative electrons into the object (positive charges in the ground). When the grounding is removed, the negative charges will be "trapped" in the object.
A positively charged rod is brought close to a neutral, grounded object. The rod is removed from the object, then the grounding is removed from the object, in that order.
Solution
When the rod is removed, since the grounding is still connected to the object, the charges redistribute evenly (neutral).
A negatively charged rod is placed in contact with a neutral, ungrounded object. The rod is removed from the object.
Solution
Placing a charged rod in contact will charge the object.
A negatively charged rod is placed in contact with a neutral, grounded object. The grounding is removed from the object, then the rod is removed from the object, in that order.
Solution
Placing a charged rod in contact will not charge the object when it is grounded. This is because the grounding can be considered as an infinite supply (or sink) of positive and negative charges, which will always make both the rod and the object uncharged (neutral) when connected and grounded.
Electric Field Lines
Electric field lines are always directed away from a positive charge and towards a negative charge.
Coulomb's Law
Coulomb's law is used to calculate the electric force between electrically charged particles.
Coulomb's law states that the electric force is proportional to the product of the charges and inversely proportional to the square of the separation distance.
Doubling the distance between two charges, while doubling the magnitude of one of the charges would result in what change to the electric force (Fe)?
Solution
The result is Fe decreases by a factor of ½.
Elementary Charge (e)
The charge on an electron is -1.6 × 10-19 C
The charge on a proton is +1.6 × 10-19 C.
Solution
The charge on a proton is equal in magnitude to the charge on an electron, with an opposite sign.
A charged object has a net charge of +2.72 × 10-18 C. Which of the following statements is true?
Solution
e = +1.6 × 10-19 C
q = +2.72 × 10-18 C
Find N
We cannot determine the total number of protons, we can only say that there are 17 more protons than electrons in this charged object.
For example, if there were 18 protons and 1 electron, the net charge would still be +2.72 × 10-18 C.
q = +2.72 × 10-18 C
Find N We cannot determine the total number of protons, we can only say that there are 17 more protons than electrons in this charged object.
For example, if there were 18 protons and 1 electron, the net charge would still be +2.72 × 10-18 C.
An unknown element has a nuclear charge of +2.24 × 10-18 C. Determine the identity of the element. Solution
| Element | Total Number of Protons |
| Carbon | 6 |
| Sodium | 11 |
| Silicon | 14 |
| Calcium | 20 |
Total Charge and Current
An object is charged with a total net of 100 electrons, given the elementary charge e = 1.6 × 10-19 C.
Find the total charge (Q).
Solution
Q =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
C
Hint Unavailable
e = -1.6 × 10-19C
Q =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
C
Hint Unavailable
Current (i) is the flow of charges per unit of time.
Calculate the time to discharge this charge if the current is 1.0 × 10-10 A.
Solution
t =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
s
Hint Unavailable
Q = = -1.6 × 10-17C
i = 1.0 × 10-10A
t =
Hint
Clear
Info
× 10
Incorrect Attempts:
CHECK
s
Hint Unavailable
i = 1.0 × 10-10A
AC and DC
Direct current is used in household wall outlets, and alternating current is used in batteries.
Solution
Other way around:
Alternating current is used in household wall outlets, and direct current is used in batteries.
Alternating current is used in household wall outlets, and direct current is used in batteries.
Naming Electrical Quantities
State the correct name when referring to the quantity:
10.0 V
Calculations with V
A 10 V battery powers a device with a 2 mA current. Calculate the energy applied over 1 minute.
Solution
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
2 mA = 2 × 10-3 A
1 minute = 60 s
W =
Hint
Clear
Info
Incorrect Attempts:
CHECK
J
Hint Unavailable
1 minute = 60 s
Conventional Current
Which direction does conventional current flow in the circuit:
Solution

Conventional current is the flow of positive charges. The longer side of the cell is positive and the positive charges flow from the positive terminal, to the negative terminal.


Series and Parallel
Which two statements are true? (check both)

The Law of Conservation of Energy
The law of conservation of energy states:
The sum of the energy gained in one circuit is equal to the sum of energy lost in one circuit.
Energy is gained in _________________, and used in _________________. (Fill in the blanks)
Energy and Volts
Voltage is also called electric potential. A cell increases electrical potential energy and a load uses electrical potential energy.
A power source (like a battery for example) creates the potential difference in a circuit.
Solution
Potential difference is the correct term for the unofficial term "voltage".
Batteries store electric potential energy per unit of charge (electron).
Batteries store electric potential energy per unit of charge (electron).
Voltage = Electromotive Force (EMF)
Solution
EMF is an outdated term. Voltage is an unofficial term. The most correct term to use in place of "voltage" and EMF is potential difference.
Voltage = Energy
Solution
This is almost true. However, voltage (electric potential) is electric potential energy, in Joules per unit of charge, q. See that voltage is J/C.
So, voltage is electric potential (and not electric potential energy).
So, voltage is electric potential (and not electric potential energy).
Circuit Types and their Equations
Match the correct equations with the type of circuits below.

- RT = R1 + R2 + ...
- VT = V1 + V2 + ...
- VT = V1 = V2 = ...
- iT = i1 + i2 + ...
- iT = i1 = i2 = ...
A series circuit uses which equations?
A parallel circuit uses which equations?
Kirchhoff's Laws
Complete the following using the laws of Gustav R. Kirchhoff (1824 - 1887).
Determine the voltage across resistor 1.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
The total increase in electric potential (voltage) is equal to the total decrease in electric potential across one path in a circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
The total increase in electric potential (voltage) is equal to the total decrease in electric potential across one path in a circuit.

Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable

Determine the voltage across resistor 2.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
The total increase in electric potential (voltage) is equal to the total decrease in electric potential across one path in a circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
The total increase in electric potential (voltage) is equal to the total decrease in electric potential across one path in a circuit.

Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable

Determine the current across resistor 1.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
The current at the start of a junction is equal to the current at the end point of that junction.
Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
The current at the start of a junction is equal to the current at the end point of that junction.

Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable

Determine the current across resistor 2.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
The current at the start of a junction is equal to the current at the end point of that junction.
Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
The current at the start of a junction is equal to the current at the end point of that junction.

Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable

Kirchhoff's Laws
Determine the missing potential differences and currents using Kirchhoff's laws.
Solution
 Hint
Clear
Info
i1 = A
Hint
Clear
Info
i1 = A
V2 = V
V3 = V
i3 = A
Incorrect Attempts:
CHECK
Hint Unavailable

Hint
Clear
Info
i1 = A
V2 = V
V3 = V
i3 = A
V2 = V
V3 = V
i3 = A
Incorrect Attempts:
CHECK
Hint Unavailable

Ohm's Law
Ohm's law can be applied as:
Kirchhoff's and Ohm's Laws
Use Kirchhoff's law and Ohm's law (below) to solve the following circuits.
V = i RA simple combination of series and parallel loads.
Solution
 Hint
Clear
Info
V1 = V
Hint
Clear
Info
V1 = V
i3 = A
i1 = A
V3 = V
R1 = Ω
R2 = Ω
R3 = Ω
Incorrect Attempts:
CHECK
Hint Unavailable

Hint
Clear
Info
V1 = V
i3 = A
i1 = A
V3 = V
R1 = Ω
R2 = Ω
R3 = Ω
i3 = A
i1 = A
V3 = V
R1 = Ω
R2 = Ω
R3 = Ω
Incorrect Attempts:
CHECK
Hint Unavailable

A more involved combination of series and parallel loads.
Solution
 Hint
Clear
Info
V2 = V
Hint
Clear
Info
V2 = V
i1 = A
i2 = A
R2 = Ω
V3 = V
V4 = V
R4 = Ω
i3 = A
iT = A
Incorrect Attempts:
CHECK
Hint Unavailable

Hint
Clear
Info
V2 = V
i1 = A
i2 = A
R2 = Ω
V3 = V
V4 = V
R4 = Ω
i3 = A
iT = A
i1 = A
i2 = A
R2 = Ω
V3 = V
V4 = V
R4 = Ω
i3 = A
iT = A
Incorrect Attempts:
CHECK
Hint Unavailable

Power
Power is the rate of energy use per unit of time.
True or false?
Which situation has the lowest power?
Solution
Magnetism
Magnetic Fields
Determine the incorrect statement about magnetic fields.
Solution
Magnetic field lines are not straight, nor are they parallel. Field lines curve in 3D space.
Simple Magnetic Induction
The north pole (N) of a permanent magnetic is placed close to an iron rod. Determine the induced magnetic poles in the iron rod at the points (1) and (2).
Solution

The north pole of the permanent magnet induces a south pole at the nearest end of the iron rod...
(1) = S and (2) = N
Magnetism Theory
Complete the following questions on the theories of magnets.
The direction of a magnetic field in a material is based on the net vector direction of the alignment of the dipoles of atoms in the material.
Solution
True.
Permanent magnets with a north (N) and south (S) pole can be permanently reversed, switching the poles.
Solution
This happens when a magnet is brought in close proximity to an even stronger magnet. The stronger magnet has a stronger magnetic field that dominates and aligns the dipole direction of the atoms in the weaker magnet. This reverses or switches the poles in the weaker magnet.
Which of the following would not demagnetize a magnet?
Solution
Breaking the magnet in half conserves the magnetic dipole, it just divides the magnet in half and makes two separate magnets.
For a certain material, a magnet can be infinitely polarized if the magnetic field is strong enough
Solution
For a certain material, a magnet can only be polarized up the point where all dipoles are aligned (in the same direction). There is a saturation point for every magnetic material where no more atomic dipoles can be aligned.
(Changing the material or increasing the size of the magnet doesn't count).
(Changing the material or increasing the size of the magnet doesn't count).
Magnets, when stored individually, lose their magnetism over time.
Solution
Kinetic molecular theory of the atoms in a magnet explains that the atomic dipoles experience internal forces that, in combination with the magnetic poles, can demagnetize the magnet over time.
(Note that magnets can be stored in a way to maintain their charge).
(Note that magnets can be stored in a way to maintain their charge).
Right Hand Rule of Electromagnetism
Determine the direction of the induced magnetic field at each point in some of the diagrams below.
The right hand grip rule is used for determining the direction of a magnetic field due to conventional current in a wire.
Solution
True. Check it out...
Conventional current is defined as the flow of positive charges.
Solution
While it is the delocalized (free) electrons in a metal that carries a current, by convention the flow of positive charges in the opposite direction is used.
A dot is used to indicate current coming out of the page, while an 'x' us used to indicate current going into the page.
Solution
If the conventional current in a current-carrying wire travels to the right, at a location above the wire the magnetic field will point:
Solution



If the conventional current in a current-carrying wire is directed into the page, at a location above the wire the magnetic field will point:
Solution



Electromagnets (Solenoids)
A solenoid is a helical coil made of a conductive material, typically copper wire.
A solenoid forms a magnetic field when current flows through the coils in an electrical circuit.
The magnitude of magnetic field is highest in what part of a solenoid
Which of the following does not increase the strength of an electromagnet?
Solution
Increasing the diameter of the coil (making a bigger coil) spreads the magnetic field lines apart, decreasing the density of the field lines, lowering the magnetic field strength.
The right hand grip rule can be used for determining the direction of magnetic fields in a solenoid.
Determine the direction of the magnetic field in the core of the solenoid. (sorry for the bad diagram)
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Use the right hand grip rule around one of the coils. (See that the current comes from the side of the source with the longer bar)
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Use the right hand grip rule around one of the coils. (See that the current comes from the side of the source with the longer bar)
To the left:

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
To the left:

Determine the direction of the magnetic field in the core of the solenoid.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Use the right hand grip rule... (See that the current comes out at the top and goes in at the bottom)
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Use the right hand grip rule... (See that the current comes out at the top and goes in at the bottom)
To the right:

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
To the right:

Force on a Current-Carrying Wire
A current carrying wire is placed in a magnetic field. Determine the direction of force.
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Conventional current points into the page. Magnetic field is always directed from N to S, which in this case is to the left. Using the left hand rule for conventional current, the force is directed upwards.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Conventional current points into the page. Magnetic field is always directed from N to S, which in this case is to the left. Using the left hand rule for conventional current, the force is directed upwards.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Magnetism: Induction
Electromagnetic Induction
Current (i) produces a magnetic field (B), and magnetic field (B) can produce a current (i).
Solution
A coil experiences an induced current when the magnetic field passing through it varies in magnitude or direction.
Moving a magnet through the middle of a coil of a conductive material will create (induce) a movement of charges (current) in that material.
Solution
True. Current is produced when the magnet is moving through the wire coil, but not produced when the magnet is stationary.
The speed of the magnet moving through a coil has no influence on the amount of current induced.
Solution
False. Moving the magnet with greater speed will induce more current. No speed (speed of zero) will induce no current.
The strength of the magnet and number of coils in the wire does not affect the amount of current induced.
Solution
False.
Lenz's Law to Determine the Direction of Induced Current
Moving a magnet through a solenoid creates a current. Determine the direction of the conventional current in the forward edge of the coil.
When the north pole of the magnet moves toward the coil...
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Get the correct current direction using the left hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves towards.

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Get the correct current direction using the left hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves towards.

When the south pole of the magnet moves toward the coil...
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Get the correct current direction using the left hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves towards.

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Get the correct current direction using the left hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves towards.

When the (closest) north pole of the magnet moves away from the coil...
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Get the correct current direction using the right hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves away.

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Get the correct current direction using the right hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves away.

When the (closest) south pole of the magnet moves away from the coil...
Solution
 Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Basically, the induced current will form in the direction that creates a magnetic field through the solenoid that repels the movement of the magnet. The energy required to push the magnet through the solenoid, against this repulsive force is inducing the energy that is "created" in the circuit.
Get the correct current direction using the right hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves away.

Hint
Clear
Info
Incorrect Attempts:
CHECK
Hint Unavailable
Get the correct current direction using the right hand grip rule (thumb = current, fingers = magnetic field) when the magnet moves away.

AC and DC Power Generation
What is the main difference between an AC and DC power generation setup?
Solution
The slip rings used in an AC generator maintain continual contact between the rotating loop and the rest of the circuit, causing an alternation in current direction.
A DC generator setup uses a split-ring commutator to connect the loop to the rest of the circuit. This maintains the same direction of current in the circuit.
A DC generator setup uses a split-ring commutator to connect the loop to the rest of the circuit. This maintains the same direction of current in the circuit.
Transformer
A physics student finds an old transformer that has 20 turns of wire on the primary (input) side and 10 turns on the secondary (induced) side. What kind of transformer is this?
Solution

The ratio of emf's in the circuits is equal to the ratio of the number of turns.
p is primary, s is secondary.
The secondary voltage is half the primary voltage. Therefore this is a step down transformer.
p is primary, s is secondary. The secondary voltage is half the primary voltage. Therefore this is a step down transformer.
The primary power equals the secondary power in all step up or step down transformers.
Solution
Transformer
A primary circuit in a step up transformer has a 120 V potential difference and 230 turns.
If the secondary circuit has 500 turns, what is the secondary voltage?
Solution
V =
Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
V is the voltage, N is the number of turns.
V =
Hint
Clear
Info
Incorrect Attempts:
CHECK
V
Hint Unavailable
If the primary current is 10 A, determine the current in the secondary circuit.
Solution
i =
Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
i =
Hint
Clear
Info
Incorrect Attempts:
CHECK
A
Hint Unavailable
